Upcoming Talks:
应用分析(applied analysis seminar)
Title: Stochastic homogenization of Hamilton-Jacobi equations: an overview of results and challenges
Time and venue: Friday, 4-5 pm, 11/29, 2024; Shuangqing C548 （updated）
Speaker: Prof. Elena KOSYGINA, NYU Shanghai & CUNY (上海纽约大学&美国纽约城市大学)
Abstract: Since the pioneering unpublished paper of P.-L. Lions, G. Papanicolaou, S.R.S. Varadhan (circa 1987), where they proposed two different approaches to the homogenization problem for Hamilton-Jacobi equations with periodic Hamitonians, the subject of homogenization of inviscid and viscous Hamilton-Jacobi equations in stationary ergodic media has received a lot of attention. In this talk I shall first give a very brief overview of the topic and then focus on our latest results and open questions. These latest results are the joint work with Atilla Yilmaz from Temple University.
Past Talks:
Title：Monotonicity Methods for Mean Field Games: A Functional Analytic Perspective
Time：周五，2024年6月21日，下午1:30-3:00
Venue: Lecture Hall B725，Shuangqing Complex Building A, Tsinghua
Speaker：Prof. Diogo Gomes ( 沙特国王科技大学 (KAUST) )
Abstract:
This presentation explores mean field games (MFGs) through the lens of functional analysis, focusing on the role of monotonicity methods in understanding their properties and deriving solutions. We begin by introducing MFGs as models for large populations of interacting rational agents, illustrating their derivation for deterministic problems. We then examine key questions of the existence and uniqueness of MFG solutions.
Monotonicity operators emerge as a central tool in our analysis. We establish the connection between monotone operators and variational inequalities, showcasing how the latter offers a flexible framework for addressing situations where traditional solutions may not exist. We then investigate the role of Hessian operators, Bregman divergence, and regularization techniques in obtaining solutions to MFGs within this framework.
Building on this foundation, we extend our discussion to the Banach space setting, examining monotone operators between a Banach space and its dual. We present existence theorems and regularization methods tailored to this context. We conclude by exploring the concept of weak-strong uniqueness, which establishes conditions under which weak and strong solutions of MFGs coincide.
Title：Global well posedness of Score-Based Generative model via Sharp Lipschitz estimate
Speaker：Zhongjian Wang 王中剑 （新加坡南洋理工大学）
Time: 2:00-3:00 pm, June 5, 2024
Venue: C548, Shuangqing Complex Building A（双清综合楼C548报告厅）
Abstract：
We establish global well-posedness and convergence of the score-based generative models (SGM) under minimal general assumptions of initial data for score estimation. For the smooth case, we start from a Lipschitz bound of the score function with optimal time length. The optimality is validated by an example whose Lipschitz constant of scores is bounded at initial but blows up in finite time. This necessitates the separation of time scales in conventional bounds for non-log-concave distributions. In contrast, our follow up analysis only relies on a local Lipschitz condition and is valid globally in time. This leads to the convergence of numerical scheme without time separation. For the non-smooth case, we show that the optimal Lipschitz bound is $O(1/t)$ in the point-wise sense for distributions supported on a compact, smooth and low-dimensional manifold with boundary.
Time: 2024/4/17, Wednesday, 2-3PM
Location: 双清综合楼B725
Speaker: Dr. Timo Sprekeler, National University of Singapore (新加坡国立大学)
Title: Homogenization of nondivergence-form PDEs with discontinuous coefficients: analysis and numerical methods
Abstract:
We study the homogenization of the PDE $-A(x/\varepsilon):D^2 u_{\varepsilon} = f$ posed in a bounded convex domain subject to a Dirichlet boundary condition and the numerical approximation of the homogenized problem, where the measurable, uniformly elliptic, periodic and symmetric diffusion matrix $A$ is merely assumed to be essentially bounded and (in dimension $n>2$) to satisfy the Cordes condition. In the first part, we show existence and uniqueness of an invariant measure and prove homogenization under minimal regularity assumptions. Then, we generalize known corrector bounds and results on optimal convergence rates from the classical case of H\"{o}lder continuous coefficients to the present case. In the second part, we discuss an approximation scheme for the effective coefficient matrix based on a finite element method for the approximation of the invariant measure.
Title: Stochastic epidemic models with memory, varying infectivity/susceptibility and waning immunity
Speaker: Guodong Pang, Rice University
Time：Tues., 2:00 pm-3:00 pm, Dec. 12, 2023
Venue：Shuangqing Complex Building 双清综合楼B627
Abstract:
In this talk we will discuss several stochastic epidemic models recently developed to account for general infectious durations, infection-age dependent infectivity and/or progress loss of immunity/varying susceptibility, extending the standard epidemic models. We construct individual based stochastic models, and prove scaling limits for the associated epidemic dynamics in large populations. Each individual is associated with a random function/process that represents the infection-age dependent infectivity force to exert on other individuals. We extend this formulation to associate each individual with a random function that represents the loss of immunity/varying susceptibility. A typical infectivity function first increases and then decreases from the epoch of becoming infected to the time of recovery, while a typical immunity/susceptibility function gradually increases from the time of recovery to the time of losing immunity and becoming susceptible. The scaling limits are deterministic and stochastic Volterra integral equations. We present some new PDEs models arising from the scaling limits. We also discuss some spatial models with varying infectivity and the associated scaling limits and PDE models. (This talk is based on recent joint work with Etienne Pardoux, Raphael Forien, and Arsene Brice Zosta Ngoufack.)
Title: Reduced models for rarefied gases and geophysical flows using moment hierarchies
Speaker: Julian Koellermeier, Bernoulli Institute, University of Groningen, the Netherlands
Time：10:00-11:30，Wednesday，Sept. 6th，2023.
Venue：YMSC双清教室B626；腾讯会议：577-4340-4459
Abstract:
The high dimension of many mathematical models in science and engineering leads to prohibitively large computational cost for even an approximate numerical solution. However, an accurate solution of the full model is often not even necessary, as only a small set of variables suffices to characterise the main behaviour of the solution. This poses the question of model reduction: How can we efficiently reduce the complexity of the model and arrive at a reduced model, that is both sufficiently accurate and computationally feasible?
In this talk, we fist briefly discuss different model reduction techniques for kinetic equations. We then focus on moment models as one way to reduce the full model to a set of analytical, lower-dimensional equations. The benefits of moment models are the mathematically sound derivation, their hierarchical structure, and the possibility to assess analytical properties of the model from the resulting equations.
We consider, among other, examples from rarefied gases and free-surface flows and illustrate those by numerical simulations.
Title: Rate of Convergence in Periodic Homogenization for Convex Hamilton-Jacobi Equations with Multiscales
Speaker: Ms. Yuxi HAN (University of Wisconsin in Madison, USA)
Time: 2023/8/8, 2:00-3:00 pm
Venue: 1st conference room, West Jin Chun Yuan Building (西楼第一会议室)
Abstract:
In practice, a wide range of problems encompasses both macroscale and microscale variables. However, our primary interest often lies in understanding the macroscale behavior. To achieve this, we employ a process known as homogenization, which aims to average out the microscale behavior. In this talk, my main focus will be on examining the rate of convergence in homogenization for convex Hamilton-Jacobi equations with multiscales. Specifically, I will demonstrate that for the Cauchy problem, the rate of convergence is O(\sqrt{\epsilon}), and the power of \epsilon is optimal. This is a joint work with Jiwoong Jang.
Title: Generalized convergence of solutions for nonlinear Hamilton--Jacobi equations with state-constraint
Speaker: Dr. Son TU (Michigan State University, USA)
Time: 10:30-11:30 am, August 3, 2023
Venue: Lecture Hall, Floor 3, Jin Chun Yuan West Bldg. (近春园西楼三楼报告厅)
Abstract:
We examine the asymptotic behaviors of solutions to Hamilton-Jacobi equations with state constraints while varying the underlying domains. We establish a connection between the convergence of these solutions and the regularity of the additive eigenvalues in relation to the domains. To accomplish this, we introduce a framework based on Mather measures that enables us to compute the one-sided derivative of these additive eigenvalues under different scenarios, including first-order, second-order, and contact first-order equations. Additionally, we provide examples of how this framework can be applied to other settings.
Title: How much can one learn a PDE from its solution?
Speaker: Prof. Yimin ZHONG (钟一民)，Auburn University, USA
Time: Fri.，2023/7/21，10:30-11:30
Venue: Lecture Hall, Floor 3, Jin Chun Yuan West Bldg.(近春园西楼三楼报告厅)
Abstract:
In this work we study a few basic questions for PDE learning from observed solution data. Using various types of PDEs, we show 1) how the approximate dimension (richness) of the data space spanned by all snapshots along a solution trajectory depends on the differential operator and initial data, and 2) identifiability of a differential operator from solution data on local patches. Then we propose a consistent and sparse local regression method (CaSLR) for general PDE identification. Our method is data driven and requires minimal amount of local measurements in space and time from a single solution trajectory by enforcing global consistency and sparsity.
Title: Optimal homogenization rates in the stochastic homogenization in a balanced random environment
Speaker: Prof. Xiaoqin GUO (郭小钦）, University of Cincinnati, USA
Time: 2023/7/4 16:20-17:20
Venue: Lecture Hall, Floor 3, Jin Chun Yuan West Bldg. (近春园西楼三楼报告厅); Zoom Meeting ID: 293 812 9202 Passcode: BIMSA
Abstract:
In this talk we consider the stochastic homogenization of elliptic non-divergence form equations on the integer lattice and the corresponding model of random walks in random environment (RWRE) which is a martingale. We will derive the optimal rates of the homogenization for the Dirichlet problem. We will also discuss the correlation structure of the invariant measure and quantitative estimates for the quenched central limit theorem of the RWRE. Joint work with Hung V. Tran (UW-Madison).
Title: Homogenization error of unsteady flow ruled by Darcy's law
Speaker: 徐强 (兰州大学)
Meeting time: （Special time!）10:00-11:00, April 15, 2022.
Zoom Meeting ID: 427 154 2002, Passcode: BIMSA
Abstract:
This talk is devoted to studying homogenization error for non-stationary Stokes equations on perforated domains, which originally developed by J.-L. Lions. We now present a sharp error estimate in the sense of energy norms, where the main challenge is to control the boundary layers caused by the incompressibility condition. To obtain the optimal error, we first introduce some refined regularity estimates for corrector without compatibility conditions between initial and boundary data, as well as, the well posedness of the effective equations in Bochner space. Then, we further explain how we handle the boundary-layer correctors associated with Bogovskii's operator. This work is cooperated with Dr. Li Wang and Prof. Zhifei Zhang in Peking University.
Title: Homogenization of elliptic systems with multiscales
Speaker: 钮维生 (安徽大学)
Meeting time (时间): 14:00-15:00, October 15, 2021.
Abstract (摘要): We review some quantitative results in the homogenization of second-order elliptic systems with periodic coefficientsthat oscillate on multiple separated scales. The results include convergence rates and some uniform regularity estimates we obtained recently.
Title: Babuska Problem in Composite Materials and Some Applications.
Speaker: 李海刚 （北京师范大学）
Meeting time: 14:00-15:00, October 22, 2021.
Abstract: In high-contrast fiber-reinforced composite materials, the stress concentration between two adjacent inclusions is a common phenomenon, which always causes damage initiation. For the original problem proposed by Ivo Babuska concerning the system of linear elasticity, we develop an iteration technique with respect to the energy integral to overcome the difficulty from the lack of maximal principle in PDE theory and obtain the blow-up asymptotic expressions of the gradients of solutions to the Lame system with partially infinite coefficients in the narrow region between inclusions when they are close to touch. Our results hold for convex inclusions with arbitrary shape and in all dimensions. As an application, we recently proved an extended Flaherty-Keller formula on the effective elastic property of a periodic composite with densely packed fibers, which is related to the “Vigdergauz microstructure” in the shape optimizition of fibers. On the other hand, we recently applied our results to deal with the resonant behavior between two close-to-touching convex acoustic subwavelength resonators.
About the speaker: 李海刚，北京师范大学教授、博士生导师，主要从事材料科学中的偏微分方程理论研究。在复合材料中的Babuska问题及其相关领域做出一系列深刻原创成果，在Adv. Math.、ARMA、JMPA、JFA等杂志发表论文30余篇。2016年获得教育部霍英东青年教师基金，2018年获得教育部自然科学二等奖，2020年入选教育部长江学者奖励计划青年学者。
Title: Global solutions of 2D isentropic compressible Navier-Stokes equations with one slow variable.
Speaker: 吕勇（南京大学）
Meeting time: 14:00-15:00, October 29, 2021.
Abstract: We prove the global existence of solutions to the two-dimensional isentropic compressible Navier-Stokes equations with smooth initial data which is slowly varying in one direction and with initial density being away from vacuum. In particular, we present examples of initial data which generate unique global smooth solutions to 2D compressible Navier-Stokes equations with constant viscosity and with initial data which are neither small perturbation of constant state nor of small energy. This talk is based on a joint paper with P. Zhang (AMSS).
Title: Layer potential approach to homogenization of sound-absorbing and resonant acoustic metamaterials
Speaker: Florian Feppon （ETH Zürich）
Meeting time: 15:00-16:00, November 12, 2021.
Abstract: In this presentation, I will present some new results about the derivation of an effective medium theory for sound-absorbing acoustic metamaterials. We determine an effective equation satisfied by the leading order asymptotic of the acoustic field scattered by many tiny obstacles filling a given volume and provide quantitative error estimates. One originality of our work is that we consider a fully non-periodic setting where the centers of the obstacles are randomly and independently distributed. Our analysis relies crucially on two new ingredients. First, using layer potentials, we show that the leading order asymptotic of the scattered field is determined by the solution to an algebraic linear system of size the number of obstacles, which can be physically interpreted as the usual ``Foldy-Lax approximation'' of the heterogeneous medium. The second ingredient is the convergence in some mean-square sense of this linear system towards a ``homogenized'' integral equation, from which the effective physics of the medium is inferred. We obtain as such that sound-absorbing media behave up to some critical size as metamaterials with positive effective refractive index. The case of high-contrast acoustic obstacles will then be briefly discussed, which lead to metamaterials exhibiting a positive or negative refractive index for an incident field whose frequency is respectively slightly smaller or slightly larger than resonant frequencies.
Title: Extrapolation, weighted Good-$\lambda$ type bounds on Lipschitz domain, with application to Homogenization of Systems of Elasticity.
Speaker: 耿俊 （兰州大学）
Meeting time: 14:00-15:00. Friday, November 19, 2021.
Abstract: For a family of systems of linear elasticity with rapidly oscillating periodic, bounded measurable coefficients, we give a sufficient condition for the weighted $W^{1,2}$ estimates for weak solutions of Neumann problems in a fixed bounded Lipschitz domains by using a weighted real variable method.
Title: A Semilinear Interface Problem Arising from Population Genetics
Meeting Time: 14:00-15:00. Friday, November 26, 2021.
Speaker: 苏琳琳 （南方科技大学）
Abstract: We study a semilinear parabolic problem that models the evolution of gene frequencies under the joint action of migration and selection with a geographical barrier. The barrier locates at an embedded interface and is of Kedem-Katchalsky type. Our main goal is to investigate the effects of the barrier on the global dynamics and on the existence, stability, and profile of spatially nonconstant equilibria.
Title: 多组分渗流数值模拟开源软件开发
Meeting Time: 15:00-16:00. Friday, November 26, 2021.
Speaker: 张晨松（中科院数学与系统科学研究院）
Abstract: 由于油气资源的成分复杂，油藏工程师在描述原油的性质时，需要使用不同的数学模型。除了常用的黑油模型之外，还有多种组分模型，如常平衡系数K模型、EOS状态方程组分模型等。一些复杂的驱油技术常基于组分模型的框架来进行数值模拟，例如聚合物驱油剂、表面活化剂驱油剂、碱驱油剂、微生物驱油剂、低盐度水驱等。一些在多孔介质中的流体运动，如二氧化碳的埋藏、地下水污染的处理、地热资源的开采等，也可使用组分模型来模拟。这些重要的应用使得组分模型逐渐成为油藏数值模拟的一个重要研究方向，但组分模型求解变量多、方程复杂，计算工作量也更大。我们基于一个通用组分模型框架，面向并行计算机体系结构，来构建快速求解方法及其开源软件平台。我们将讨论多组分渗流力学的工业应用、物理背景、数学模型、离散方法、快速求解、并行计算及其软件开发。
About the speaker: 张晨松，2002年获南京大学计算数学硕士学位，2007年获得美国马里兰大学应用数学博士学位，此后在宾州州立大学数学系从事博士后研究工作，于2011年进入中国科学院数学与系统科学研究院计算数学所，任副研究员。主要研究兴趣：自适应有限元方法、多层迭代法及其在流固耦合、油藏模拟等问题中的应用。2012年在第二十一届国际区域分解法会议（法国）做大会特邀报告，2013年在第16届全国流体力学数值方法会议做大会特邀报告。曾在Numer Math、SINUM、SIAM MMS、M3AS、JCP、Handbook of Numerical Analysis等国际一流期刊发表了多篇学术论文。
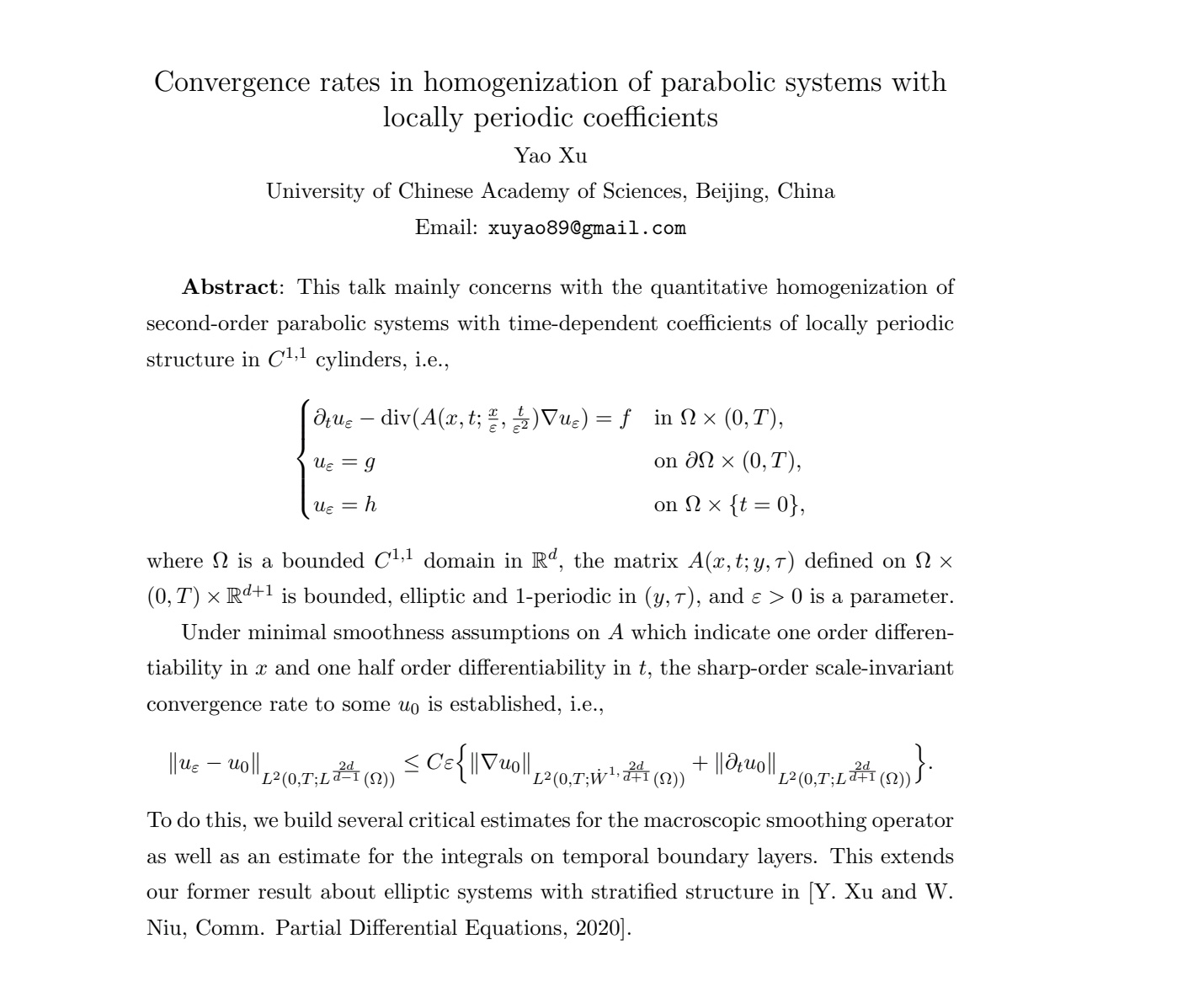
Title: Convergence rates in homogenization of parabolic systems with locally periodic coefficients.
Meeting Time: 14:00-15:00. Friday, December 3, 2021.
Speaker: 徐侥 （中国科学院大学）
摘要: 见图中信息。
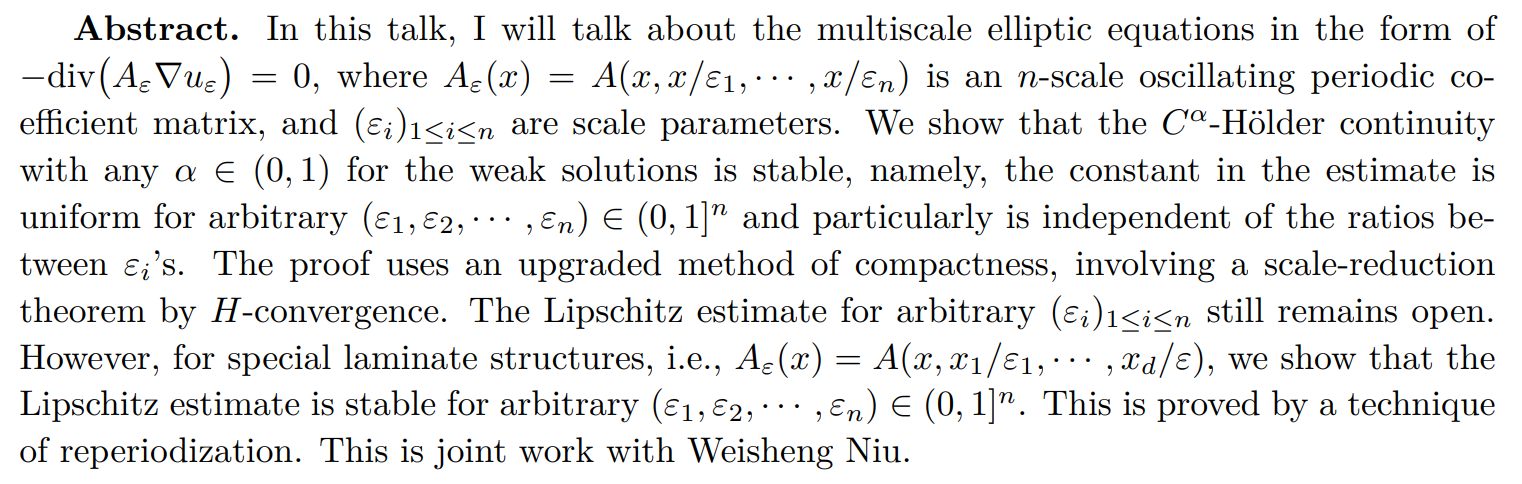
Title: Compactness and stable regularity in multi-scale homogenization.
Meeting time：(Special) 中午12:00-13:00, Friday, December 17, 2021
Speaker: Jinping Zhuge （The University of Chicago）
摘要：见图中信息。
Title: Some recent developments on numerical homogenization
Meeting time: 14:00-15:00. Friday, December 31, 2021.
线上：Zoom Meeting ID: 361 038 6975, Passcode: BIMSA
Zoom link: https://zoom.us/j/98193841924?pwd=L2p5cTgxU1ZLUGRucllFSWNlZkl2UT09
Speaker: 张镭 （上海交通大学）
Abstract:Problems with a wide range of coupled temporal and spatial scales are ubiquitous in many phenomena and processes of materials science and biology. Numerical homogenization concerns the approximation of the high dimensional solution space of multiscale PDEs by a low dimensional approximation space with optimal error control, and furthermore, the efficient construction of such an approximation space, e.g., the localization of the basis on a coarse patch. There has been a vast amount of work concerning the design and analysis of numerical homogenization type methods for multiscale problems, such as asymptotic homogenization, numerical upscaling, heterogeneous multi-scale methods, multi-scale finite element methods, variational multi-scale methods, flux norm homogenization, rough polyharmonic splines (RPS), generalized multi-scale finite element methods, localized orthogonal decomposition, etc.Surprisingly, numerical homogenization has deep connections with Bayesian inference, kernel learning and probabilistic numerics. The Bayesian homogenization approach provides a unified framework for the construction of a proper coarse space with desired approximation and localization properties, and practically it corresponds to a variational problem with functional constraints, which is close to in this sense.In this talk, I will give a brief introduction of the recent development in numerical homogenization, its connection with Bayesian inference, fast solvers, and machine learning. I will also discuss some possible further directions.
Title: Homogenization of Linear Elliptic Equations in Nondivergence-Form: Characterizations of Good Diffusion Matrices
Meeting Time: 14:00-15:00. Friday, January 7, 2022.
线上：Zoom Meeting ID: 361 038 6975, Passcode: BIMSA
Speaker: Timo Sprekeler （National University of Singapore）
Abstract: In this talk, we discuss the periodic homogenization of linear elliptic equations of the form $-A(x/\varepsilon):D^2 u^{\varepsilon} = f$ subject to a Dirichlet boundary condition. We characterize good diffusion matrices $A$, i.e., those for which the sequence of solutions converges at a rate of $\mathcal{O}(\varepsilon^2)$ in the $L^{\infty}$-norm to the solution of the homogenized problem. Such diffusion ma/trices are considered “good” as the optimal rate of convergence in the generic case is only $\mathcal{O}(\varepsilon)$. First, we provide a class of good diffusion matrices, confirming a conjecture posed by Guo and Tran in 2020. Then, we give a complete characterization of diagonal diffusion matrices in two dimensions and a systematic study in higher dimensions. This talk is based on joint work with Xiaoqin Guo (University of Cincinnati) and Hung V. Tran (University of Wisconsin Madison).
Title: Stochastic homogenization for reaction-diffusion equations and G-equations
Meeting time: 14:00-15:00, March 4, 2022.
Speaker: Yuming Paul Zhang (University of California - San Diego)
Abstract: Homogenization is a general phenomenon when physical processes in periodic or random environments exhibit homogeneous long time dynamics due to large space averaging of the variations in the environment. While this area of Mathematics saw a slew of remarkable developments in the last 20 years, the progress in the case of reaction-diffusion equations, which model many important physical phenomena, has been somewhat limited due to the homogenized dynamic involving discontinuous solutions to different (first-order) equations. In this talk I will discuss the first proofs of homogenization for reaction-diffusion equations with random not necessarily isotropic reactions in several spatial dimensions. These include the cases of both time-independent and time-dependent reactions, with the later proof employing a new subadditive ergodic theorem for time-dependent environments. We obtain analogous results for G-equations with random flame speeds and incompressible background advections.
This talk is based on joint works with Andrej Zlatoš.
Title: 近壁湍流有界吗？
Meeting time: (Special time)10:30-11:30, March 18, 2022.
Speaker: 陈曦 （北航大学)
摘要: 探索湍流的终极统计不变性(the ultimate statistical invariance of turbulence)是一个经典的科学问题。特别是对于自然界和工业界广泛存在的湍流边界层而言，因为流体的能量主要在近壁面被耗散掉，因此预测近壁湍流在无穷大雷诺数时的渐近态具有重要的科学和工程意义。一个世纪前，Prandtl提出了经典的壁面律（Law-of-wall）理论并在平均流速的预测上获得极大成功，但是对于湍流的脉动平均量所言有限。最近六十年的实验和计算数据显示，多达二十余项的近壁湍流脉动量（如脉动能极值、压力极值、涡量极值、耗散极值、扩散极值、速度高阶矩极值，以及壁面剪应力和壁面压力等等，通记为Φ）随着雷诺数（Re）增加而显著增长。对此，学术界一个普遍性的观点认为Φ∝ln(Re)，意味着随着Re增加Φ将发散性增长从而壁面律将失效。与此不同的是，本报告将介绍本人最近发展的有限耗散率理论（Law-of-bounded-dissipation）以及普适雷诺数相似律：Φ∞-Φ∝Re-1/4，新的结论是在Re→∞时诸Φ各为其有限Φ∞所界（见Chen & Sreenivasan, 2021 JFM; 2022 JFM）。目前，这一观点引发了国际学者的密切关注和讨论 (如：Monkewitz 2022 JFM；Smits et al 2021 JFM；Pirozzoli et al 2021 JFM)，相关进展在此也将有所介绍。
